算額（その962）

算額（その962）

一七 大里郡岡部村岡 稲荷社 文化13年(1816)

埼玉県立図書館：埼玉県史料集 第二集『埼玉の算額』，昭和44年，誠美堂印刷所，埼玉県与野市.

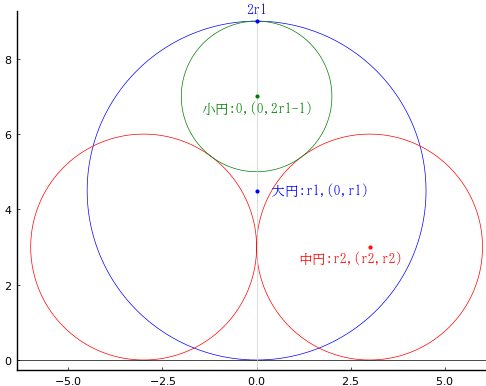
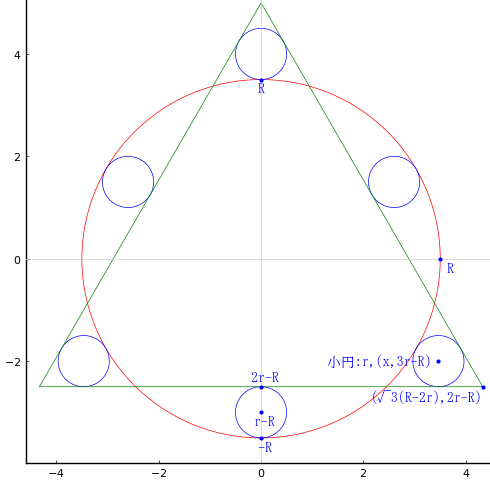
直線上に中円 2 個が互いに接して載っており，その上に小円が載っている。大円も直線上にあり，小円が内接している。大円，中円の直径がそれぞれ 9 寸，6 寸のとき，小円の直径はいかほどか。

大円の半径と中心座標を r1, (0, r1)
中円の半径と中心座標を r2, (r2, r2)
小円の半径と中心座標を r3, (0, r1 - r3)
とおき，以下の方程式を解く。

include("julia-source.txt");

using SymPy
@syms r1::positive, r2::positive, r3::positive
eq1 = r2^2 + (2r1 - r3 - r2)^2 - (r2 + r3)^2
res = solve(eq1, r3)[1]
res |> println

   (2*r1 - r2)^2/(4*r1)

小円の半径 r3 は，大円の半径 r1，中円の半径 r2 により，(2r1 - r2)^2/4r1 で求めることができる。

大円，中円の直径がそれぞれ 9 寸，6 寸のとき，小円の直径は 2(2r1 - r2)^2/4r1 = 4 寸である。

r1 = 9/2
r2 = 6/2
2(2r1 - r2)^2/4r1

   4.0

function draw(more=false)
   pyplot(size=(500, 500), grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
   (r1, r2) = (9, 6) ./ 2
   r3 = (2*r1 - r2)^2/(4*r1)
   @printf("大円の直径が %g, 中円の直径が %g のとき，小円の直径 = %g\n", 2r1, 2r2, 2r3)
   plot()
   circle(0, r1, r1, :blue)
   circle2(r2, r2, r2)
   circle(0, 2r1 - r3, r3, :green)
   if more        
       delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
       hline!([0], color=:black, lw=0.5)
       vline!([0], color=:gray80, lw=0.5)
       point(0, r1, "  大円:r1,(0,r1)", :blue, :left, :vcenter)
       point(r2, r2, "中円:r2,(r2,r2)", :red, :center, delta=-delta, deltax=-4delta)
       point(0, 2r1 - r3, "小円:0,(0,2r1-1)", :green, :center, delta=-delta)
       point(0, 2r1, "2r1", :blue, :center, :bottom, delta=delta)
   end
end;

算額（その961）

算額（その961）

一七 大里郡岡部村岡 稲荷社 文化13年(1816)

埼玉県立図書館：埼玉県史料集 第二集『埼玉の算額』，昭和44年，誠美堂印刷所，埼玉県与野市.

正方形内に半円を 4 個入れ，交差した部分に等円を 4 個入れる。正方形の一辺の長さが 41 寸のとき，等円の直径はいかほどか。

正方形の一辺の長さを 2a
等円の半径と中心座標を r, (x, x); x = a/2
とおき，以下の連立方程式を解く。

include("julia-source.txt");

using SymPy
@syms a::positive, r::positive, x::positive
x = a/2
eq1 = (x - a)^2 + x^2 - (a - r)^2
res = solve(eq1, r)[1]
res |> println

   a*(2 - sqrt(2))/2

等円の直径 2r は正方形の一辺の長さ 2a の (2 - √2)/2 倍である。
正方形の一辺の長さが 41 寸のとき，等円の直径は 41*(2 - √2)/2 = 12.00862197135155 寸である。

41*(2 - √2)/2

   12.00862197135155

function draw(more=false)
   pyplot(size=(500, 500), grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
   a = 41/2
   x = a/2
   r = a*(2 - √2)/2
   @printf("正方形の一辺の長さ = %g;  等円の直径 = %g\n", 2a, 2r)
   plot([a, a, -a, -a, a], [-a, a, a, -a, -a], color=:green, lw=0.5)
   circle(a, 0, a, beginangle=90, endangle=270)
   circle(0, a, a, beginangle=180, endangle=360)
   circle(-a, 0, a, beginangle=-90, endangle=90)
   circle(0, -a, a, beginangle=0, endangle=180)
   circle4(x, x, r, :blue)
   if more        
       delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
       hline!([0], color=:gray80, lw=0.5)
       vline!([0], color=:gray80, lw=0.5)
       point(a, 0, " a", :green, :left, :vcenter)
       point(0, a, " a", :green, :center, :bottom, delta=delta/2)
       point(x, x, "等円:r,(x,x)", :blue, :center, delta=-delta/2)
   end
end;

算額（その960）

算額（その960）

一七 大里郡岡部村岡 稲荷社 文化13年(1816)

埼玉県立図書館：埼玉県史料集 第二集『埼玉の算額』，昭和44年，誠美堂印刷所，埼玉県与野市.

大小の正方形と正三角形を積み重ねている。大きな正方形の一辺の長さが 112 寸のとき，小さい正方形の一辺の長さを求めよ。

大小の正方形の一辺の長さを a, b，正三角形の一辺の長さを c として，以下の方程式を解く。
eq1 は線分の長さの関係，eq2 は面積を 2 通りの方法で計算して等しいと置くものである。

include("julia-source.txt");

using SymPy
@syms a::positive, b::positive, c::positive
eq1 = a + b/2 - c√Sym(3)/2
eq2 = (a + b + c)*(a + b)/2 - (a^2 + b^2 + (c * c/2*√Sym(3) + a^2/√Sym(3) + b^2/√Sym(3)/2)/2)
res = solve([eq1, eq2], (a, c))[1]

   (-b/2 + sqrt(3)*(-b/2 - b/(sqrt(3) + 3) + 7*sqrt(3)*b/6)/2, -b/2 - b/(sqrt(3) + 3) + 7*sqrt(3)*b/6)

数式は簡約化できる。

res[1] |> simplify |> println
res[2] |> simplify |> println

   -sqrt(3)*b/2 + 3*b/2
   -b + 4*sqrt(3)*b/3

小正方形の一辺の長さは大正方形の一辺の長さの (3 - √3)/2 倍，
正三角形の一辺の長さは大正方形の一辺の長さの (4√3/3 - 1) 倍である。

b = 112
b*(3 - √3)/2  |> println
b*(4√3/3 - 1) |> println

   71.00515477614287
   146.65292059695233

大正方形の一辺の長さが 112 寸のとき，小正方形の一辺の長さは 71.00515477614287 寸，
正三角形の一辺の長さは 146.65292059695233 寸である。

function draw(more=false)
   pyplot(size=(500, 500), grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
   b = 112
   a = b*(3 - √3)/2 
   c = b*(4√3/3 - 1)
   @printf("a = %g;  c = %g\n", a,  c)
   plot([0, √3c/2, 0, 0], [a, a + c/2, a + c, a], color=:green, lw=0.5)
   rect(0, 0, a, a, :blue)
   rect(a, 0, a + b, b, :red)
   segment(√3c/2, a + c/2, a + b, b, :green)
   if more        
       delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
       hline!([0], color=:gray80, lw=0.5)
       vline!([0], color=:gray80, lw=0.5)
       point(a, 0, "a", :blue, :center, delta=-delta/2)
       point(a + b, 0, "a+b", :red, :center, delta=-delta/2)
       point(0, a, "a ", :blue, :right, :vcenter)
       point(0, a + c, "a+c ", :blue, :right, :vcenter)
       point(√3c/2, a + c/2, "(√3c/2,a+c/2)", :green, :left, :bottom, delta=delta/2)
       plot!(xlims=(-6delta, a + b + 3delta), ylims=(-3delta, a + c + 3delta))
   end
end;

算額（その959）

算額（その959）

一七 大里郡岡部村岡 稲荷社 文化13年(1816)

埼玉県立図書館：埼玉県史料集 第二集『埼玉の算額』，昭和44年，誠美堂印刷所，埼玉県与野市.

外円の中に，正方形と甲円，乙円が入っている。外円の直径が 475 寸のとき，乙円の直径はいかほどか。

正方形の一辺の長さを 2a
外円の半径と中心座標を R, (0, 0)
甲円の半径と中心座標を r1, (0, R - r1); r1 = a
乙円の半径と中心座標を r2, (r2 - a, r2 - a)
とおき，以下の連立方程式を解く。

include("julia-source.txt");

using SymPy
@syms R::positive, a::positive, r1::positive, r2::positive
a = R/√Sym(2)
r1 = a
eq1 = (r2 - a)^2 + (R - r1 - r2 + a)^2 - (r1 + r2)^2
res = solve(eq1, r2)[2]
res |>  println

   R*(-sqrt(2 + 2*sqrt(2)) + 1 + sqrt(2))

乙円の半径は外円の半径の (1 + √2 - sqrt(2 + 2√2)) 倍である。
外円の直径が 475 寸のとき，乙円の直径は 475(1 + √2 - sqrt(2 + 2√2) = 103.00153433280056 寸である。

475*(1 + √2 - sqrt(2 + 2√2))

   103.00153433280056

なお，正方形の一辺の長さは外円の半径の √2 倍，甲円の直径は正方形の一辺の長さに等しい。

r2 = 51.5008;  R = 237.5;  a = 167.938;  r1 = 167.938

475/2 * √2

   335.8757210636101

function draw(more=false)
   pyplot(size=(500, 500), grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
   R = 475/2
   r2 = R*(-sqrt(2 + 2*sqrt(2)) + 1 + sqrt(2))
   a = R/√2
   r1 = a
   @printf("外円の直径が %g のとき，乙円の直径は %g である。\nr2 = %g;  R = %g;  a = %g;  r1 = %g\n", 2R, 2r2, r2, R, a, r1)
   plot([a, a, -a, -a, a], [-a, a, a, -a, -a], color=:blue, lw=0.5)
   circle(0, 0, R)
   circle(0, R - r1, r1, :green)
   circle(r2 - a, r2 - a, r2, :magenta)
   if more        
       delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
       hline!([0], color=:gray80, lw=0.5)
       vline!([0], color=:gray80, lw=0.5)
       point(a, a, " (a,a)", :blue, :left, :vcenter)
       point(0, R - r1, "甲円:r1,(0,R-r1)", :green, :center, delta=-delta/2)
       point(r2 - a, r2 - a, "乙円:r2\n(r2-a,r2-a)", :magenta, :center, :bottom, delta=delta/2)
       point(0, R, " R", :red, :center, :bottom, delta=delta/2)
   end
end;

算額（その958）

算額（その958）

一七 大里郡岡部村岡 稲荷社 文化13年(1816)

埼玉県立図書館：埼玉県史料集 第二集『埼玉の算額』，昭和44年，誠美堂印刷所，埼玉県与野市.

大小 2 個の正方形が交差している。区分された領域に甲円 1 個，乙円 2 個を入れる。甲円の直径が 577 寸のとき，乙円の直径はいかほどか。

大きい正方形の一辺の長さを a
小さい正方形の一辺の長さを c
小さい正方形の頂点と大きい正方形の一辺の交点座標を (b, 0), (0, b)
甲円の半径と中心座標を r1, (r1, r1)
乙円の半径と中心座標を r2, (a + r2, c/√2)
とおき，以下の連立方程式を解く。

include("julia-source.txt");

using SymPy
@syms a::positive, b::positive, r1::positive, r2::positive
c = √Sym(2)a - b/√Sym(2)
eq1 = 2b - c - 2r1
eq2 = r1/r2 - c/b
eq3 = 2b^2 - c^2
res = solve([eq1, eq2, eq3], (r2, a, b))[1]

   (sqrt(2)*r1/2, 3*r1*(sqrt(2) + 2)/2, r1*(sqrt(2) + 2))

乙円の半径は甲円の半径の √2/2 倍である。
甲円の直径が 577 寸のとき，乙円の直径は 577*√2/2 = 408.000612744638 寸である。

r1 = 577/2
(r2, a, b) = (3*r1*(sqrt(2) + 2)/2, r1*(sqrt(2) + 2), sqrt(2)*r1/2)
577*√2/2

   408.000612744638

function draw(more=false)
   pyplot(size=(500, 500), grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
   r1 = 577/2
   (r2, a, b) = (sqrt(2)*r1/2, 3*r1*(sqrt(2) + 2)/2, r1*(sqrt(2) + 2))
   c = √2a - b/√2
   @printf("a = %g;  b = %g;  c = %g;  r2 = %g;  2r2 = %g\n", a, b, c, r2, 2r2)
   plot([0, a, a, 0, 0], [0, 0, a, a, 0], color=:blue, lw=0.5)
   plot!([b, b + c/√2, b, 0, b], [0, c/√2, 2c/√2, b, 0], color=:green, lw=0.5)
   circle(r1, r1, r1)
   circle(a + r2, c/√2, r2, :magenta)
   if more        
       delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
       hline!([0], color=:gray80, lw=0.5)
       vline!([0], color=:gray80, lw=0.5)
       point(a, 0, " a", :blue, :center, delta=-delta)
       point(0, a, "a ", :blue, :right, :vcenter)
       point(b, 0, " b", :blue, :center, delta=-delta)
       point(0, b, "b ", :blue, :right, :vcenter)
       point(r1, r1, "甲円:r1,(r1,r1)", :red, :center, delta=-delta)
       point(a + r2, c/√2, "乙円:r1\n(a+r2,c/√2)", :magenta, :center, :bottom, delta=delta/2)
       point(b, 2c/√2, "(b,2c/√2)", :green, :center, :bottom, delta=delta)
       plot!(xlims=(-5delta, 2c/√2+5delta), ylims=(-5delta, 2c/√2+5delta))
   end
end;

算額（その957）

算額（その957）

一七 大里郡岡部村岡 稲荷社 文化13年(1816)

埼玉県立図書館：埼玉県史料集 第二集『埼玉の算額』，昭和44年，誠美堂印刷所，埼玉県与野市.

大円と正三角形が交差している。区画された領域に小円を 7 個入れる。大円の直径が 7 寸のとき，小円の直径はいかほどか。

大円の半径と中心座標を R, (0, 0)
小円の半径と中心座標を r, (0, r - R), (x, 3r - R)
とおき，以下の連立方程式を解く。
正三角形の一辺の長さは 4(R - 2r) である。大円の中心と正三角形の頂点までの距離は 2(R - 2r) である。

include("julia-source.txt");

using SymPy
@syms R::positive, r::positive, x::positive
eq1 = r/((R - 2r)*√Sym(3) - x) - 1/√Sym(3)
eq2 = x^2 + (3r - R)^2 - (R + r)^2
res = solve([eq1, eq2], (r, x))[1]

   (R/7, 4*sqrt(3)*R/7)

小円の半径 r は大円の半径 R の 1/7 である。
したがって，大円の直径が 7 寸のとき，小円の直径は 1 寸である。

function draw(more=false)
   pyplot(size=(500, 500), grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
   R = 7/2
   (r, x) = (R/7, 4*sqrt(3)*R/7)
   @printf("小円の直径 = %g\n", 2r)
   plot()
   circle(0, 0, R)
   rotate(0, r - R, r, :blue)
   rotate(x, 3r - R, r, :blue)
   l = 2(R - 2r)
   c30 = l*cosd(30)
   s30 = l*sind(30)
   plot!([c30, 0, -c30, c30, c30],
         [-s30, l, -s30, -s30], color=:green, lw=0.5)          
   if more        
       delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
       hline!([0], color=:gray80, lw=0.5)
       vline!([0], color=:gray80, lw=0.5)
       point(0, 2r - R, " 2r-R", :blue, :center, :bottom, delta=delta/2)
       point(0, r - R, " r-R", :blue, :center, delta=-delta/2)
       point(0, -R, " -R", :blue, :center, delta=-delta/2)
       point(0, R, "R", :blue, :center, delta=-delta/2)
       point(R, 0, " R", :blue, :left, delta=-delta/2)
       point(x, 3r - R, "小円:r,(x,3r-R) ", :blue, :right, :vcenter)
       point(√3(R-2r), 2r-R, "(√3(R-2r),2r-R)", :blue, :right, delta=-delta/2)
       plot!(ylims=(-R - 4delta, 2(R - 2r) + delta))
   end
end;