算額（その362）

算額（その362）

山形県上山市金山峠 檜下不動堂
山形算額勝負-湯殿山神社を目指せ-
https://www.sci.yamagata-u.ac.jp/wasan/pdf/20180711SSEP.pdf

円内に同じ大きさの正方形が3個入っている。円の直径が 10 寸のとき，正方形の一変の長さを求めよ。

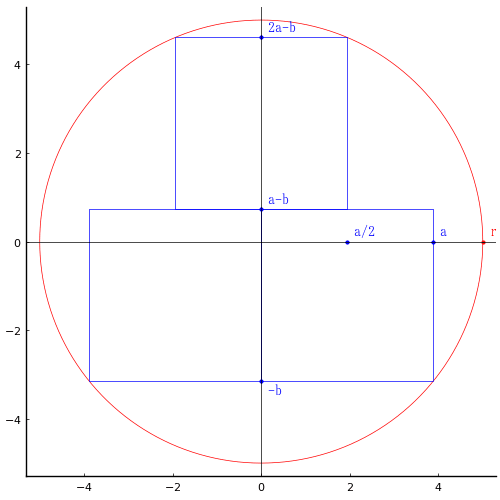
円の半径を r，正方形の一辺の長さを a とする。下にある正方形の下辺の y 座標を -b とする。
以下の連立方程式を解く。

include("julia-source.txt");

using SymPy

@syms r::positive, a::positive, b::positive;

eq1 = a^2 + b^2 - r^2
eq2 = (a/2)^2 + (2a - b)^2 - r^2;
res = solve([eq1, eq2], (a, b))

   1-element Vector{Tuple{Sym, Sym}}:
    (5*sqrt(17)*(-13*sqrt(17)*r/85 + r)*(13*sqrt(17)*r/85 + r)/(16*r), 13*sqrt(17)*r/85)

a は簡約化される。
a = 16*sqrt(17)*r/85

a = res[1][1] |> simplify
a |> println

   16*sqrt(17)*r/85

r = 10/2 のとき，a = 3.880570000581328 である。

r = 10/2
16*sqrt(17)*r/85

   3.880570000581328

using Plots

function draw(more)
    pyplot(size=(500, 500), grid=false, aspectratio=1, label="", fontfamily="IPAMincho")
   r = 10/2
   (a, b) = (5*sqrt(17)*(-13*sqrt(17)*r/85 + r)*(13*sqrt(17)*r/85 + r)/(16*r), 13*sqrt(17)*r/85)
   @printf("a = %g;  b = %g\n", a, b)
   plot()
   circle(0, 0, r)
   rect(0, -b, a, a - b, :blue)
   rect(0, -b, -a, a - b, :blue)
   rect(-a/2, a - b, a/2, 2a - b, :blue)
   if more
       delta = (fontheight = (ylims()[2]- ylims()[1]) / 500 * 10 * 2) /3  # size[2] * fontsize * 2
       point(0, -b, " -b", :blue, delta=-delta/2)
       point(r, 0, " r", :red, :left, :bottom, delta=delta/2)
       point(a, 0, " a", :blue, :left, :bottom, delta=delta/2)
       point(a/2, 0, " a/2", :blue, :left, :bottom, delta=delta/2)
       point(0, a - b, " a-b", :blue, :left, :bottom, delta=delta/2)
       point(0, 2a - b, " 2a-b", :blue, :left, :bottom, delta=delta/2)
       hline!([0], color=:black, lw=0.5)
       vline!([0], color=:black, lw=0.5)
   else
       plot!(showaxis=false)
   end
end;